Tractogram Atlasing

Limits of Standard Methods

Numerous classes of methods exist to perform spatial registration of medical data. They range from very simple linear transformations suited to small deformations to more complex non linear settings such as the Large Deformation Diffeomorphic Metric Mapping able to model more involved registrations.

Example of small-deformation based registration of a hand — The upper-left hand is registrated onto the bottom-left hand using a small-deformation based method. The different colmuns showcase the effect of an hyperparameter of the method (from low values to high): here the size of the kernel upon which the vector-field of landmarks momentum is convoluted.

Those methods are robust and widely used for instance for MRI image (T1, T2, ...) registration. They often enforce a high regularity of the resulting deformation field. This reflects anatomical priors underlying the observed image. Indeed, such deformations should not introduce wholes or anatomically incorrect effects in the images. While this increases the robustness of the registration, those assumptions might not be suited when non homeomorphic changes appear in images (such as tumors) and quickly reaches their limits when applied on geometric data such as anatomical fibers.

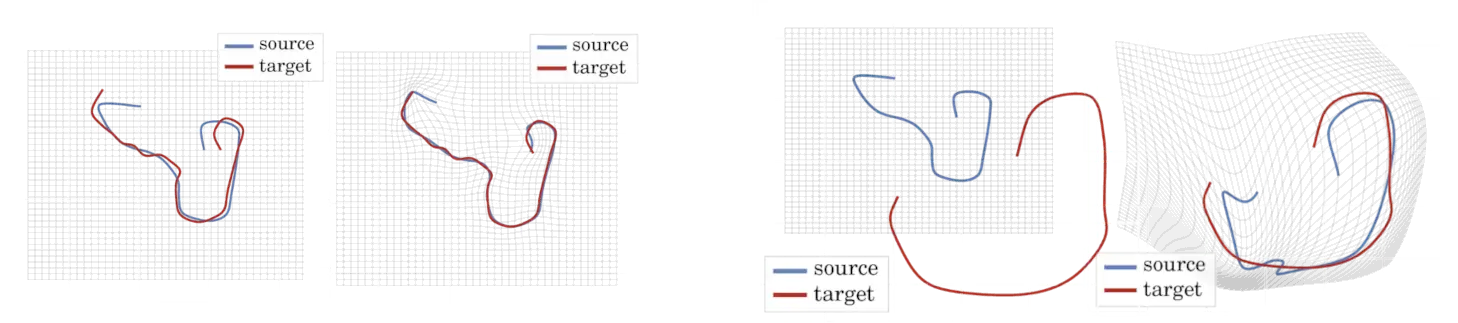
Example of a LDDMM registration of curves without topology break — Two example of curve registration where no topology rupture are needed to correctly match the curves. Here I used the LDDMM framework with a varifold metric.

Example of a LDDMM registration of curves with a topology break — Conservation of topology during registration. The left problem is easy, since no topology rupture is needed to match the curves. The right problem is harder because the ideal registration would involve to un-cross the curves, which is not allowed in the LDDMM framework used here, hence the weird result.

Solutions

Appendix: overview of all anatomical bundles considered

You can click on a bundle to observe the fibers composing it (segmented on a Human Connectome Project tractogram).

Corpus Callosum	Forceps Major	Forceps Minor
Left IFOF	Right IFOF	Left Cingulum1
Right Cingulum1	Left Cingulum2	Right Cingulum2
Left Cingulum3	Right Cingulum3	Left SFOF
Right SFOF	Left Uncinate	Right Uncinate
Left Arcuate	Right Arcuate	Left cortico-spinal
Right cortico-spinal	Left anterior thalamic	Right anterior thalamic
Left inferior thalamic	Right inferior thalamic	Left superior thalamic
Right superior thalamic	Left posterior thalamic	Right posterior thalamic
Left VOF	Right VOF	Left AntVOF
Right AntVOF	Left VisionU	Right VisionU
Right OccipitalU	Left Thalamico-spinal	Right Thalamico-spinal
Left pArc	Right pArc	Left TPC
Right TPC	Left MdLF-SPL	Right MdLF-SPL
Left MdLF-Ang	Right MdLF-Ang	Left SLF1
Right SLF1	Left SLF2	Right SLF2
Left SLF3	Right SLF3	Left ILF
Right ILF	Left Aslant	Right Aslant
Right Lenti2ceb	Left Lenti2ceb	Right Red2Ceb1
Left Red2Ceb1	Right Red2Ceb2	Left Red2Ceb2
Right Thal2ceb	Left Thal2ceb	Right ContraCorticoPontine
Left ContraCorticoPontine	Right ContraFrontoPontine	Left ContraFrontoPontine
Right IpsiCorticoPontine	Left IpsiCorticoPontine	Right IpsiFrontoPontine
Left IpsiFrontoPontine	Left Meyers Loop	Right Meyers Loop
Left Baums Loop	Right Baums Loop	Left Optic Unclassified
Right Optic Unclassified	Left Optic Accesory	Right Optic Accesory